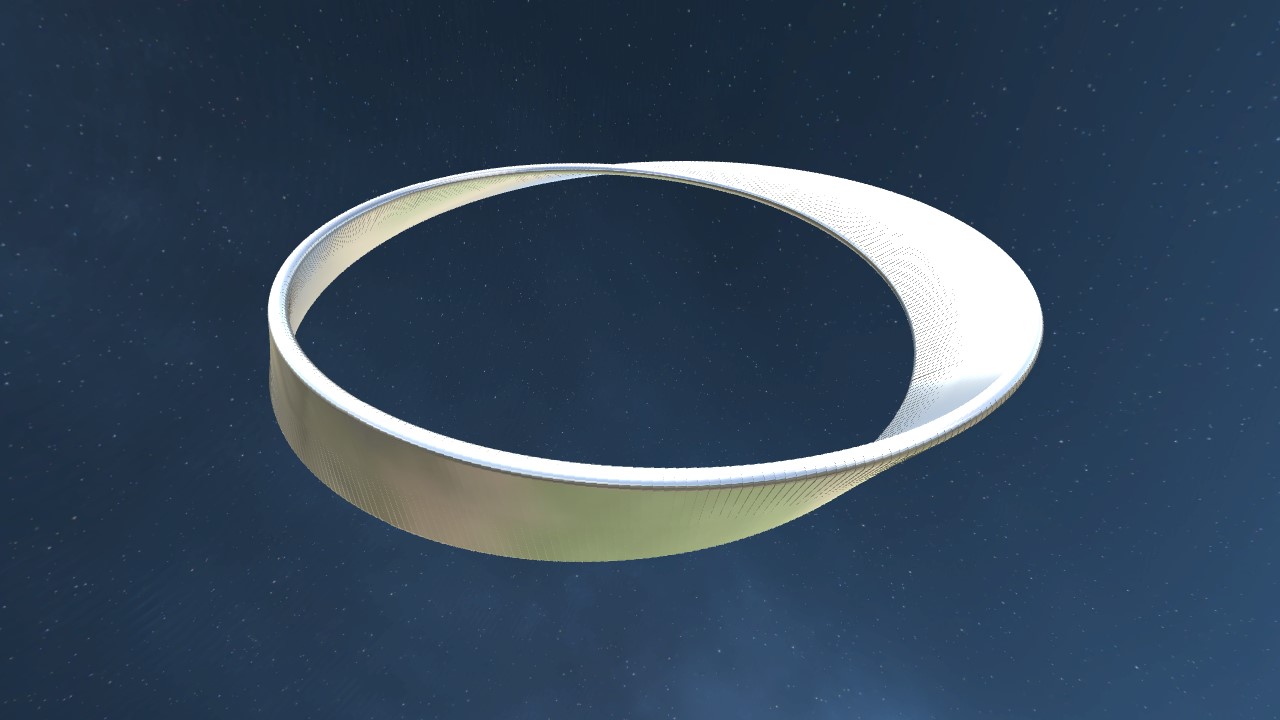
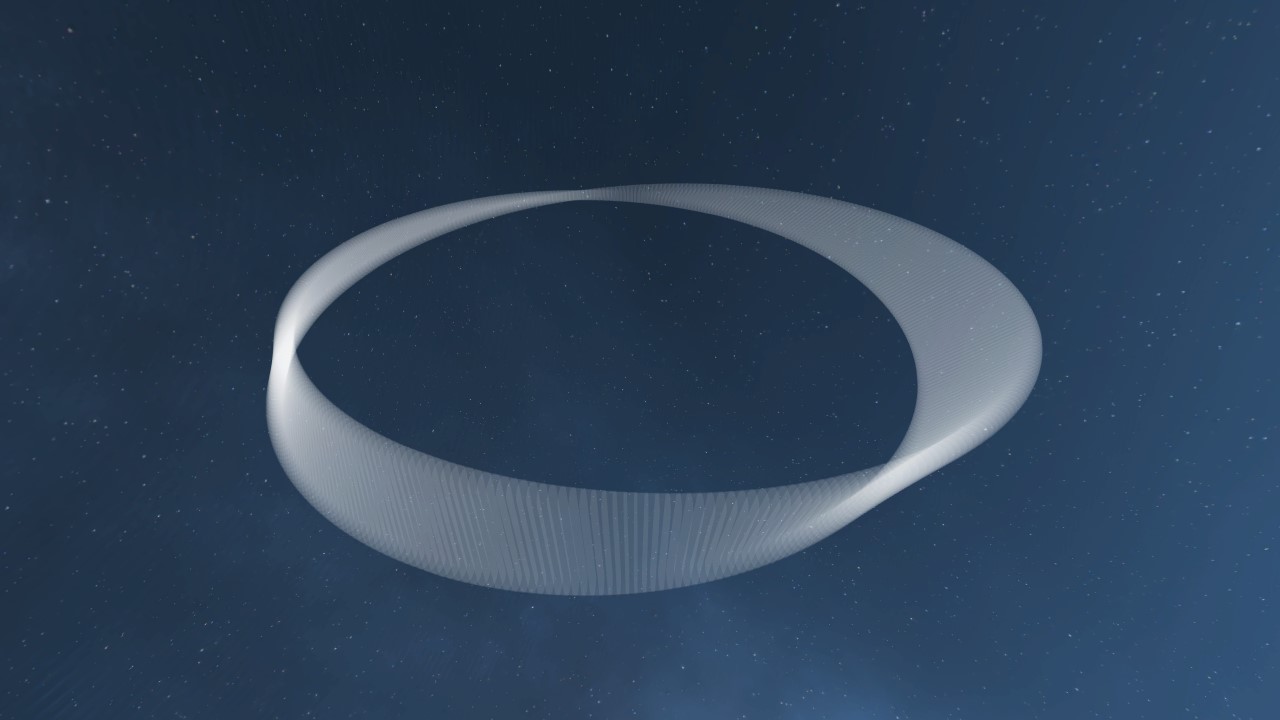
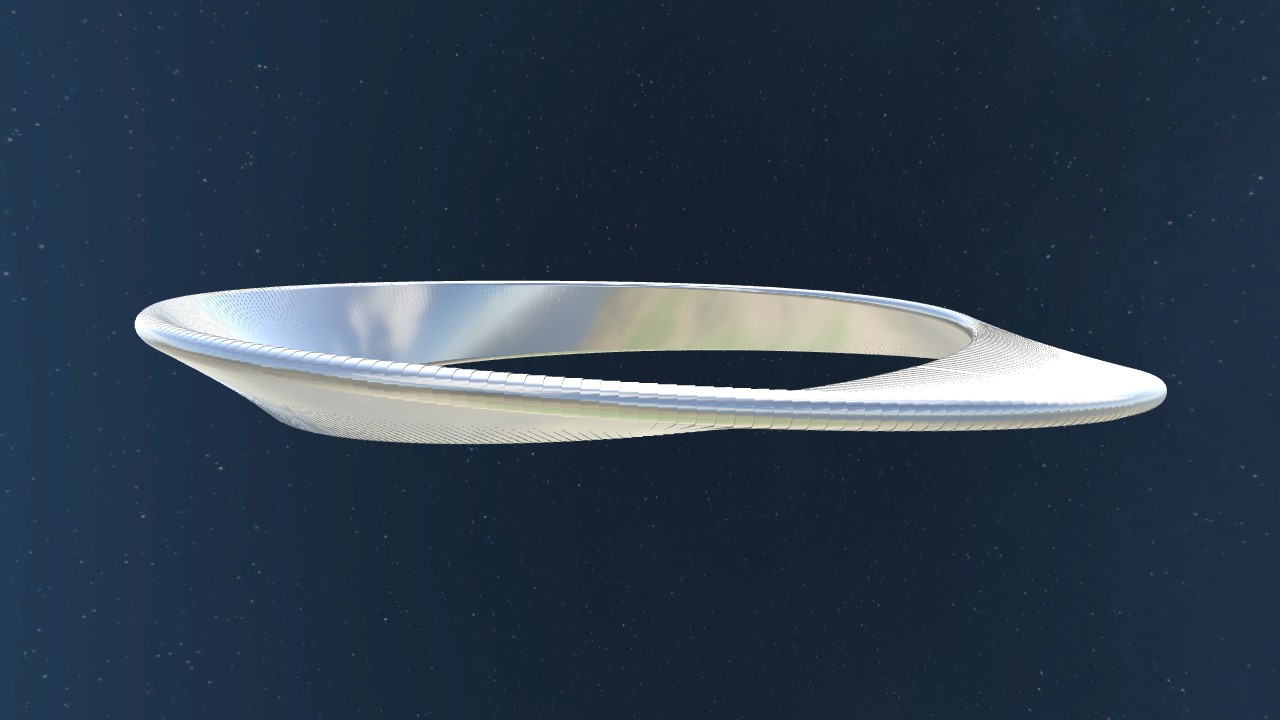
The Möbius strip or Möbius band ( (non-rhotic) or ; German: ['mø?bi??s]), also spelled Mobius or Moebius, is a surface with only one side (when embedded in three-dimensional Euclidean space) and only one boundary. The Möbius strip has the mathematical property of being unorientable. It can be realized as a ruled surface. It was discovered independently by the German mathematicians August Ferdinand Möbius and Johann Benedict Listing in 1858.

An example of a Möbius strip can be created by taking a paper strip and giving it a half-twist, and then joining the ends of the strip to form a loop. However, the Möbius strip is not a surface of only one exact size and shape, such as the half-twisted paper strip depicted in the illustration. Rather, mathematicians refer to the closed Möbius band as any surface that is homeomorphic to this strip. Its boundary is a simple closed curve, i.e., homeomorphic to a circle. This allows for a very wide variety of geometric versions of the Möbius band as surfaces each having a definite size and shape. For example, any rectangle can be glued to itself (by identifying one edge with the opposite edge after a reversal of orientation) to make a Möbius band. Some of these can be smoothly modeled in Euclidean space, and others cannot.

A half-twist clockwise gives an embedding of the Möbius strip different from that of a half-twist counterclockwise – that is, as an embedded object in Euclidean space, the Möbius strip is a chiral object with right- or left-handedness. However, the underlying topological spaces within the Möbius strip are homeomorphic in each case. An infinite number of topologically different embeddings of the same topological space into three-dimensional space exist, as the Möbius strip can also be formed by twisting the strip an odd number of times greater than one, or by knotting and twisting the strip, before joining its ends. The complete open Möbius band is an example of a topological surface that is closely related to the standard Möbius strip, but that is not homeomorphic to it.