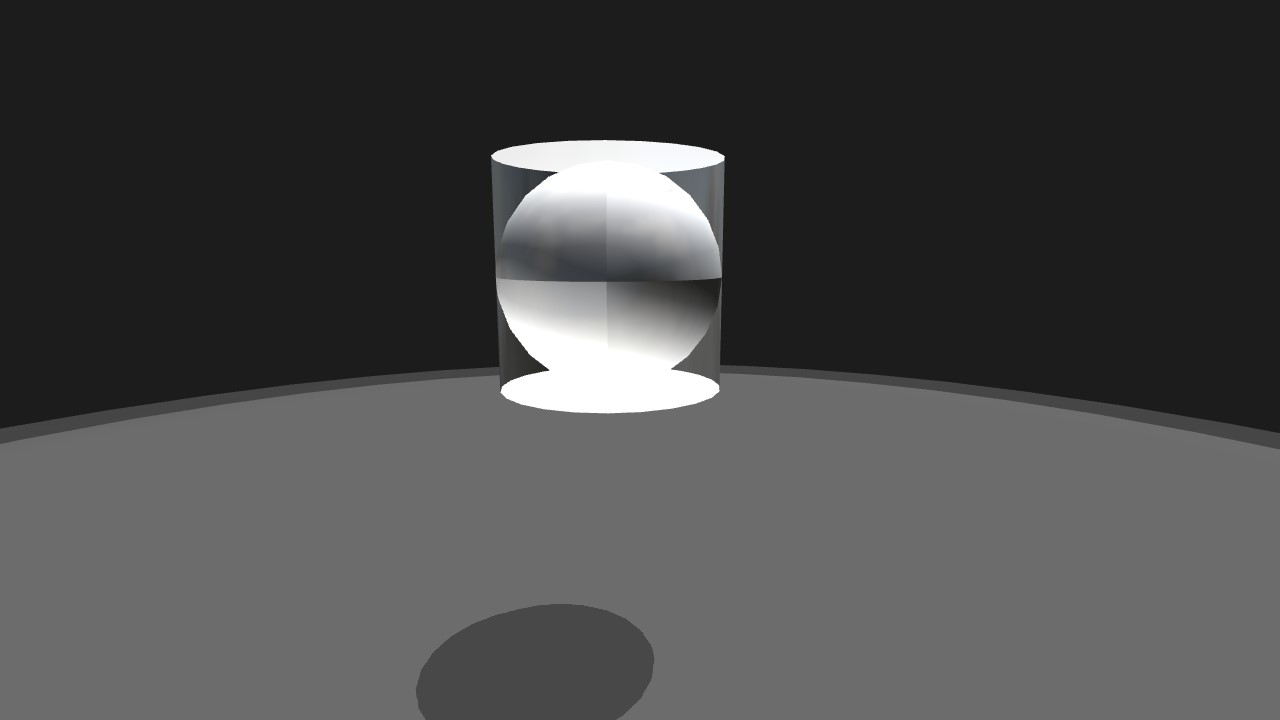
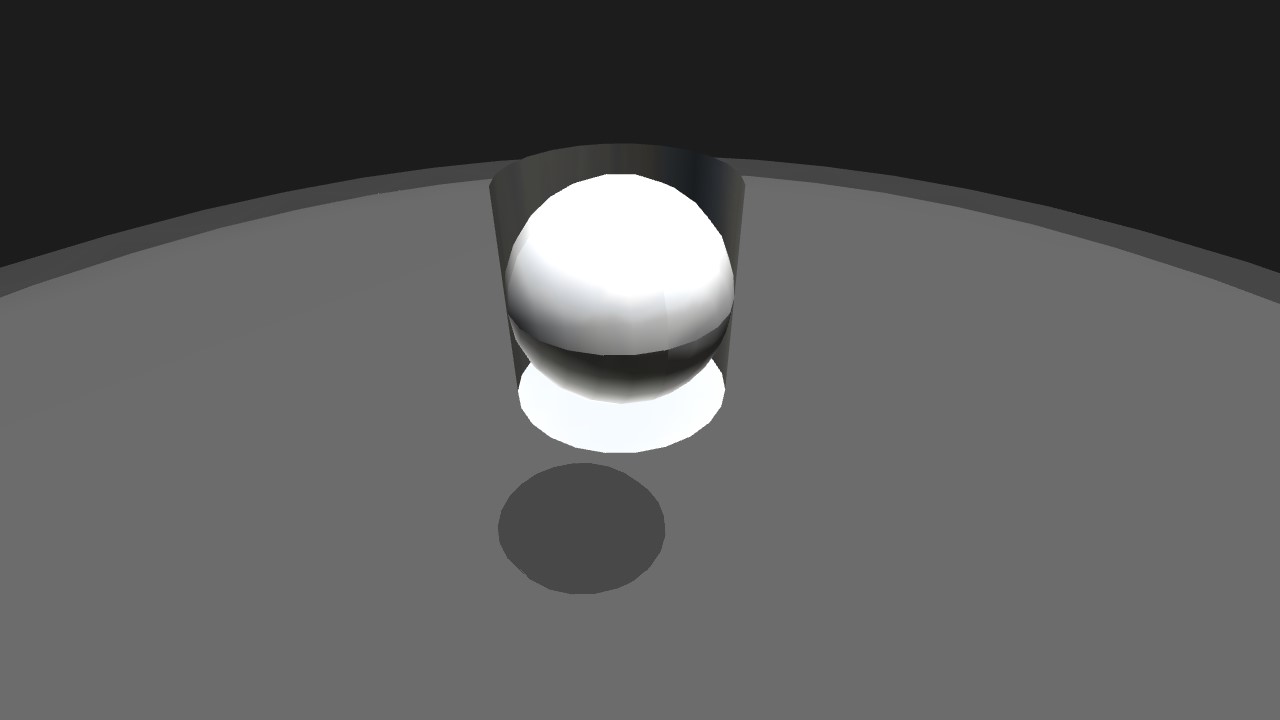
This is considered one of Archimedes greatest discoveries: The volume of an inscribed sphere is 2/3 that of the cylinder.
This is what the model above is showing.
The cylinder’s diameter is 1 meter, or 3.3 feet. I will be using meters for this.
The cylinders radius, therefore, is 0.5m.
To find the volume of the cylinder in question, we first need to find the base.
The formula to find the base is pr^2
Since the radius is 0.5m, the formula is p(0.5)^2
This comes out to be p(0.5)^2=0.785^2
Now we multiply the area of the base by the height to get the volume of the cylinder. The height is 1m
This calculation would be 0.785^2 x 1=0.79m^3
The volume of the inscribed sphere is 2/3 the volume of the cylinder
Therefore, the next operation needed is 0.66 x 0.79m^3
0.66 x 0.79m^3=0.463m^3
Thus, the volume of our inscribed sphere is 0.463m^3
What you see above is what Archimedes said was one of his greatest discoveries: The volume of a sphere is 2/3 the volume of a cylinder it would fit in perfectly. That is what my model shows. A sphere inscribed in a cylinder. I hope you enjoyed this simple geometry and I might do the Pythagorean Theorem next.
P.S. Please alert me to any errors in my calculations