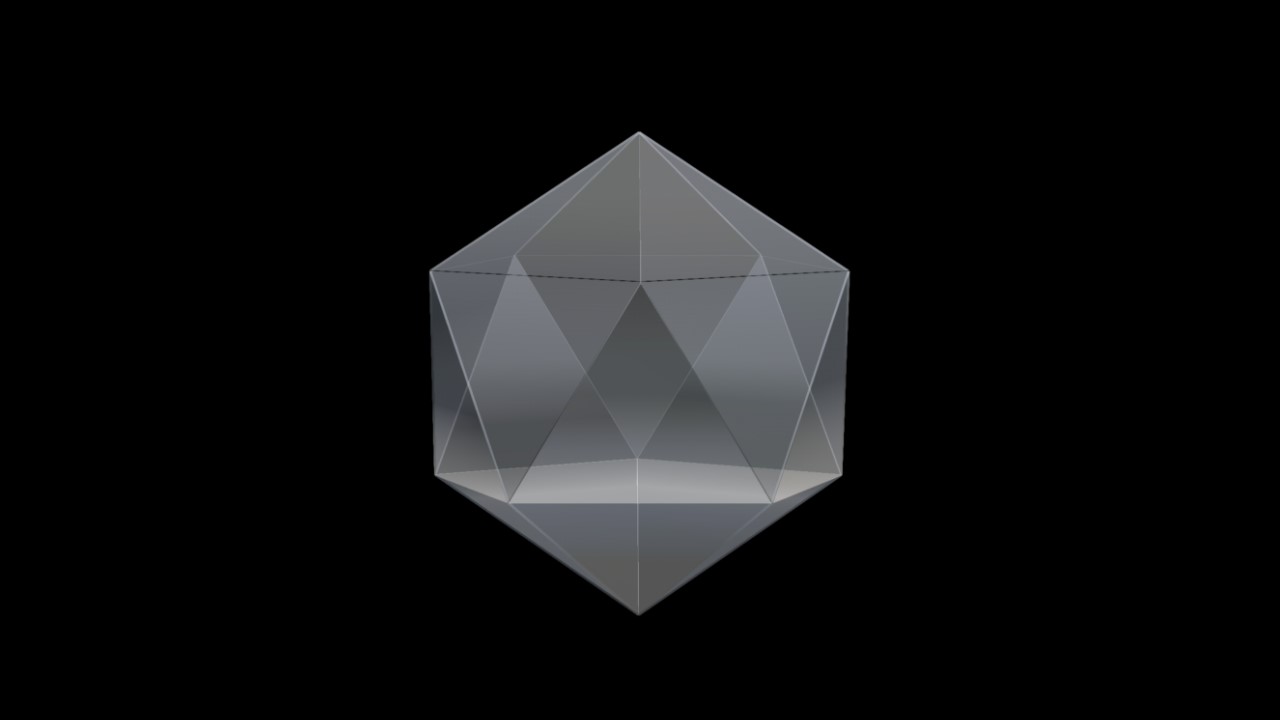
An icosahedron is a polyhedron with 20 faces. In particular, this is the regular, convex, non-stellated icosahedron, which is one of the Platonic solids, and is composed of 20 equilateral triangles. Link to Wikipedia article here.
The model provided uses equilateral triangles with a side length of 1 fuselage unit.
This model was constructed by hand using some math.
- The top "pentagon" was constructed by calculating the apothem of the orthographic projection of the pentagon, using that as a leg for a theoretical triangle between the pentagon projection and an inclined triangle, leading to the angle 37.38º. Link to math here.
- I used two of the previous "pentagon" bits with one on top and one on the bottom. Now I had to fill in the sides, which meant I had to calculate the inclination angle for the side triangle panels. I again constructed a theoretical triangle between the top pentagon and bottom pentagon. There was a horizontal difference which came down to the difference of the pentagon's circumradius and apothem, which gave me an angle of 10.81º from the normal for the side triangles.
- I had to also figure out the block positions, which took most of my time actually. It was mostly figuring out the position of the vertices and using those to calculate the position for other bits. Link to math here.
TL;DR: annoying trigonometry to figure out block positions.
I was originally trying to create buckminsterfullerene but this looked easier to start with so I took around an hour and a half to build this. It looks nice, sort of like one of those "power crystal" things in games.
Hope this is somehow useful, have fun!
- Snow
Specifications
Spotlights
- BaconAircraft 4.6 years ago
- Vincent 4.6 years ago
- 232287168147825 4.6 years ago
General Characteristics
- Successors 1 airplane(s) +28 bonus
- Created On Mac
- Wingspan 2.7ft (0.8m)
- Length 2.8ft (0.9m)
- Height 3.1ft (1.0m)
- Empty Weight 551lbs (250kg)
- Loaded Weight 551lbs (250kg)
Performance
- Wing Loading N/A
- Wing Area 0.0ft2 (0.0m2)
- Drag Points 51
Parts
- Number of Parts 1
- Control Surfaces 0
- Performance Cost 132





bacteriophage time
The quality of my IPad shows it as a square
surreal memes
@LieutenantSOT A real shape lol
@asteroidbook345 what the hell is that!? Craziness XD
The Icosahedron is the 5th Platonic Shape. Beautiful shape here
@MAHADI Oh
@CrimsonOnigiri that's because it's not connected to the cockpit
the best post with the highest intellectual comments I have read in an eon.
I wish Albert could have seen this.
you should work more on the flight model. I suggest you increase throttle range in order to reduce its acceleration while also reducing the drag via xml. Aditionally a good idea would be to keep the wing loading realistic if you want an acceptable flight behaviour.
Custom control surfaces would have been also nice
1 part!?!?!?
@Grob0s0VBRa meth too
It’s not just megamind, it’s gigamind
I’ll upvote just for your maths and the painful rotations and nudging it must have taken
@SnoWFLakE0s Popcorn not well seasoned. Too bland. Needs more salt.
@spefyjerbf
.
Indeed entertaining. I think I've provided a lot of people with decent popcorn.
@spefyjerbf To continue,
.
In high speed flows, the kinetic energy per unit mass (V^2/2), is very large which is substantial enough to strongly interact with the other properties of the flow. Since the science of energy and entropy is the thermodynamics, it is essential to study the thermodynamic aspects of gases under the conditions compressible high speed flows. A gas is considered as a collection of particles (molecules, atoms, ions, electrons etc.) that are in random motion under certain intermolecular forces. These forces vary with distances and thus influence the microscopic behavior of the gases. However, the thermodynamic aspect mainly deals with the global nature of the gases. Over wide ranges of pressures and temperatures in the compressible flow fields, it is seen that the average distance between the molecules is more than the molecular diameters (about 10-times). So, all the flow properties may be treated as macroscopic in nature. A perfect gas follows the relation of pressure, density and temperature in the form of the fundamental equation.
p = \rhoRT; R=R/M (4.1.1)
Here, M is the molecular weight of the gas, R is the gas constant that varies from gas
to gas and R (= 8314 J kg.K) is the universal gas constant.
Such an elegant way to build something.
Why eyeballing the shape when you have math in your tool box lol.
@AN2Felllla @SnoWFLakE0s @BaconAircraft Reading this comments section was the perfect way to entertain myself while on the toilet after a long day of manufacturing for my student org, so ty.
.
Just to clarify, I am mediocre at math, but every once in a while I can grasp a particularly useful concept - hence my portfolio of verbose descriptions
.
Anyway, let’s get back to the topic at hand. In general, the liquids and gases are the states of a matter that comes under the same category as “fluids”. The incompressible flows are mainly deals with the cases of constant density. Also, when the variation of density in the flow domain is negligible, then the flow can be treated as incompressible. Invariably, it is true for liquids because the density of liquid decreases slightly with temperature and moderately with pressure over a broad range of operating conditions. Hence, the liquids are considered as incompressible. On the contrary, the compressible flows are routinely defined as “variable density flows”. Thus, it is applicable only for gases where they may be considered as incompressible/compressible, depending on the conditions of operation. During the flow of gases under certain conditions, the density changes are so small that the assumption of constant density can be made with reasonable accuracy and in few other cases the density changes of the gases are very much significant (e.g. high speed flows). Due to the dual nature of gases, they need special attention and the broad area of in the study of motion of compressible flows is dealt separately in the subject of “gas dynamics”. Many engineering tasks require the compressible flow applications typically in the design of a building/tower to withstand winds, high speed flow of air over cars/trains/airplanes etc. Thus, gas dynamics is the study of fluid flows where the compressibility and the temperature changes become important. Here, the entire flow field is dominated by Mach waves and shock waves when the flow speed becomes supersonic. Most of the flow properties change across these waves from one state to other. In addition to the basic fluid dynamics, the knowledge of thermodynamics and chemical kinetics is also essential to the study of gas dynamics.
Obviously, there are many situations where more than three forces (or in fact only two forces) will be acting on a body. An alternative method is required to solve such problems.
If all forces under consideration are acting in the same plane, then these forces and the resultant force can each be expressed as a sum of its i- and j-components.
If a force F acts at an angle of θ to the x-axis, then F can be written as the sum of two forces, one ‘horizontal’ and the other ‘vertical’:
F=|F|cosθi+|F|sinθj
The force F is resolved into two components:
the i-component is parallel to the x-axis
the j-component is parallel to the y-axis.
remember this babe? @SodiumIodide
@AN2Felllla It's really not extensively lengthy. Rather than complaining about it and making futile attempts at mockery, I'd advise you to actually learn the vocabulary.
dice
Magnificent creation!
@AN2Felllla
.
Please, I challenge you, point out where I have been needlessly verbose. If you can simplify my words without changing the meaning or making it too long, I'll gladly accept the proposition that I'm doing this verbose thing intentionally.
.
I forgot to mention, please drop the openly mocking attitude. It helps no-one.
@SnoWFLakE0s It's not your math, it's the
e x t e n s i v e l yl e n g t h yv o l c a b u l a r yyou haveu t i l i s e d